A-periodic tiles are sets of 3-8 tiles that are specially shaped so that no matter what way they are joined they produce a pattern that never repeats. It seems counterintuitive to have a small set of regular shapes that can only make irregular shapes when joined. There is probably a mathematical proof for this but I have been trying to force a pattern, and disprove the theory, for long enough today to admit defeat. These shapes provide the potential in architecture is to create irregular structures from regular members; a building like the Watercube could be developed without needing to custom manufacture every joint and member.
The facade of Federation Square in Melbourne is made from pinwheel tiling. Just five regular shapes make the irregular pattern on the building.
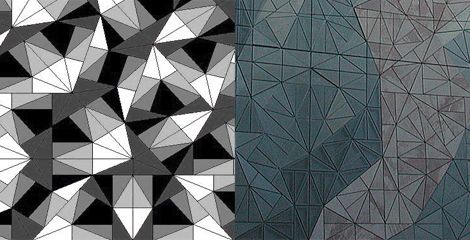
Pinwheel tiling on the left (Source: Wikipedia), the same shapes are used in Federation Square, shown on the right (Source: Wolfram).
Marc Fornes' creations use three-dimensional aperiodic tiling. While they look chaotic and irregular, if you look at his CNC cutting templates they are comprised from regular shapes. I am not sure of the exact tiling he uses but a basic version is the Danzer tiles.
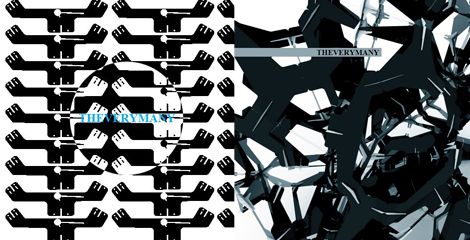
The regular shapes cut out on the left, make the irregular form on the right using 3d a-periodic tiling. Source: The Very Many.